I’m putting together slides for a TED audition talk in a couple of weeks, about how the history of quantum mechanics is like a crossword puzzle. This involves talking about black-body radiation, which is the problem that kicked off QM– to explain the spectrum of light emitted by hot objects, Max Planck had to resort to a mathematical trick: he assumed that the objects were composed of “oscillators” that emitted light in discrete amounts, with the energy of the emitted light proportional to the frequency of the light. This was a desperation move, and made him a little crazy:

(Not really, but that picture (from here) is so unlike the old-bald-dude-with-glasses image that is the default Planck picture that I love sharing it. I also like this 1878 picture of Planck quite a bit…)
Anyway, Planck’s formula kicked off the quantum revolution. Five years later (or so), Einstein ran with the idea of light quanta, and eight years after that Bohr proposed his model of hydrogen, with atoms absorbing and emitting light in discrete chunks with the frequency determined by Planck’s rule. Planck was never all that happy with the trick, but as the famous cartoon says, it works brilliantly.
Thinking about this, coupled with my usual desire to procrastinate, got me wondering about something I’ve never entirely understood, though. And while I could probably Google up an explanation somewhere, it’s probably worth a blog post to explain what I managed to distract myself with.
So, the issue is this: since the 1850’s, we’ve known that atoms absorb and emit light at discrete frequency. Bohr provided the conceptual framework for understanding this in 1913 and Schödinger, Heisenberg, and Dirac provided the mathematical machinery for predicting the characteristic frequencies of a particular atom. We understand where these frequencies come from, and can predict them to very high precision.
Meanwhile, Planck’s formula gives a broad, continuous spectrum, which again we can predict to exceptional precision, and this matches reality. The problem I have is understanding how to connect these two. That is, the objects emitting a broad spectrum of thermal radiation in keeping with Planck’s formula are made up of atoms, which we know emit light at discrete frequencies. So, how does the narrow emission of atoms get turned into the broad emission of objects?
If you’re talking about things like the filament of an incandescent bulb, one of the canonical examples of black-body radiation used in popular discussions, I’m usually okay with writing this off as one of those black-magic effects you get when you start combining excessive numbers of atoms– the solid lattice offers a crystal structure that can soak up energy, so you get inelastic processes where you absorb one frequency and emit another, and wave hands madly, broad spectrum output.
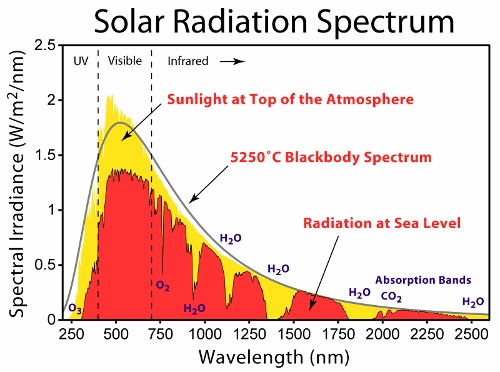
But then there’s the Sun, which is pretty close to a black-body emitter (see the spectrum in the “featured image” up top, which I got from here). The Sun isn’t a solid– like the song says, it’s a mass of incandescent gas, without a lattice structure to do black-magic things. But there again, there’s an out– it’s not really a mass of incandescent gas, it’s mostly a miasma of light-emitting plasma, with lots of free charges running around, so again, wave hands madly, broad spectrum output.
But, of course, actual neutral clouds of gas also emit thermal radiation, and people can and do use this to monitor the temeprature of gases emitted from power plants and that sort of thing. And there, you don’t really have any outs. You don’t have a solid lattice or free charges to do black magic, just a bunch of atoms that want to absorb and emit light in specific, very narrow spectral lines, and yet the end result of all this is a broad thermal spectrum of light. And I’ve never really understood how that works out at a microscopic level.
I assume that the answer is inelastic light scattering– that is, while the most direct form of interaction with radiation that AMO people like myself like to think about involves the absorption and emission of well-defined frequencies, there’s also a probability of off-resonant scattering. This is completely negligible compared to the resonant scattering that we deal with when we start blasting things with lasers, but in the absence of light deliberately tuned to resonance, it’s the only game in town. So an atom that really wants to absorb some narrow line still has a tiny probability of an absorb-and-emit interaction with photons that are way, way off that resonance, and sometimes that process will transfer energy between atoms and light. A moving atom exposed to incoming photons at one frequency will send out photons of a slightly lower frequency, speeding up a tiny bit to account for the energy difference. The probability of this happening is tiny, for any given atom, but when you start thinking about molar quantities of gas, that still adds up to a whole lot of scattering. And enough of this will eventually give you a thermal distribution.
I think that’s really the only thing that can be going on, but I’m really just guessing, here. I’ve never seen the details worked out, though I assume somebody must have thought carefully about this at some point. Anyway, I thought I’d throw this out there, on the off chance that any of my wise and worldly readers know of a great explanation of this floating around somewhere.
(Also, while the academic politics stuff has been great for blog traffic, I feel a little guilty about the lack of front-page physics content, and this fixes that problem…)
I see someone is a TMBG fan.
A cloud of gas on earth is probably full of diatomic molecules with rotational and vibrational modes that broaden transitions. But an interstellar cloud of monoatomic hydrogen would pose no such complications. Not sure what to say there.
I’ve understood it to be Bremsstrahlung from the atomic collisions, during which you can’t assume a neutral little ball. But I’ve never run though the math to see if that actually works; my exposure was a qualitative explanation in a QM text.
One of the things that set this off was a passing mention in a book about neutrinos about the time required for photons created near the core of the Sun to reach the surface. This said something about how the fusion reaction produces gamma rays, but by the time they get out a few hundred thousand years later, these have thermalized to fit a black-body spectrum at a much lower temperature. Which is why I was thinking in terms of inelastic light scattering.
Bremsstrahlung during collisions never would’ve occurred to me. I suppose you could get something like that, though. And I guess this is another place where the plasma physics hand-wave might separate the two cases.
I assume that relative motion and collisions is the result, going from fairly monochromatic mercury and low pressure sodium lights to the broader spectrum of high pressure sodium. Considering it takes many many thousands of years for photons to make it out of the sun, a lot of broadening would result.
Prof. Orzel,
Thermal collisions in gases and plasmas broaden the absorption and emission lines far beyond the natural widths. In solids or liquids the same effect arises, as you say, from the vibrations of the atoms or molecules. You can also picture this as broadening of the natural lines by collisions with phonons, lattice vibrations, which have a black body spectrum themselves.
Mybe the answer is that it many cases it doesn’t look like a blackbody, and that’s why 1850’s guys could invent spectroscopy.
A cloud of neutral gas in interstellar space doesn’t consist of 100% neutral atoms: some fraction of those atoms get ionized by passing photons. I’m not sure how large this fraction is, but it doesn’t have to be all that large to get collective effects: the ionospheric E region is ~99% neutral, but it’s still a plasma. Thus the effects that are at work in the sun are in play (though less effective) here.
Also, if you are dealing with temperatures of a few hundred kelvin or lower, the frequencies of interest are much lower than the Lyman and Balmer lines. So effects like hyperfine splitting and Zeeman splitting (there are magnetic fields around) come into play, contributing to a much broader spectrum than Balmer’s formula would lead you to expect. As you get warmer, collisional ionization and thermal speed increase, so you get plasma effects as well as Doppler broadening.
Hi Chad,
I remember a paper in the American Journal of Physics discussing this question – “Hot gases: The transition from line spectra to thermal radiation”, March 2005 (DOI 10.1119/1.1819931). When reading the paper, I did realize that I had not even know that there was something I had not understood before 😉
It’s generally a combination of line-emission and Bremsstrahlung, with Bremsstrahlung dominating at high temperatures (about more than 10^6 K).
Bernard is correct. The process of changing energy states is not unique to electron orbitals.
Quantization of angular momentum for all objects results in energy exchanges (including photon emission) on the order of Planck’s constant, a very, very small amount of angular momentum (or energy per amount of frequency). Summing up all these possible vibrational energy transitions in a system is how we can theoretically derive the radiation formula that Planck discovered empirically.
Fun fact: Planck tried very hard to take the limit of his empirical formula as his constant went to 0, but could achieve no sensible result.
My understanding is that Plank’s law is itself descended from the Rayleigh-Jeans law. I don’t have Rayleigh’s original paper to hand, but from my recollection, this introduced the transition from discrete to continuous frequencies.
In his paper deriving the asymptotic 2f^2 kT /c^2 black body approximation at low frequencies, Rayleigh considers radiation in a box, and restricts the frequencies to a discrete distribution of wave numbers (n_1,n_2,n_3).
However, in order to integrate over all integers, he then makes the step of approximating the sum of the discrete functions over n by a continuous integral over dn. The method gives the right answer, but for somewhat “wrong” reasons(Mathematically it is fine; I can’t speak for the “natural philosophy” side of it). After this point, everyone seems to have taken the wavenumbers/frequencies to be a continuous distribution instead of a discrete one.
The paper Stefan mentions in #9 looks really good, and includes a simulation of a sample of two-level “atoms” showing a transition to a broad emission line as the optical depth increases. I haven’t had time to dig into the details, but it’s now on the list of things to read in detail.
I remembered your articles on laser-cooled atoms, and thought about the relative velocities of the gas particles. How much of a contribution would these velocities have, relative to the experimenter, on broadening the emission spectra?
The thing is, the usual way of deriving the Planck spectrum that I’ve seen in my stat mech text (for example) is to consider a container with perfectly conducting walls, so that the electric field will have nodes on the walls. Those boundary conditions give standing waves in the container, and then you use Planck’s assumption that the waves have discrete, evenly-spaced energies.
Nothing in that recipe makes an assumption about the wall material, except that it should be a conductor. I always assumed you could re-solve the problem with a finite resistivity and it would just cancel out at some point in the math.
All of that is for a cavity, not a solid surface, or a liquid surface, but I know you also get the same spectrum in those cases without the standing wave picture that goes with the cavity example. (My thermal IR camera works nicely with water, based on the Stefan-Boltzmann law, which is Planck’s spectrum integrated over wavelength. Useful in the kitchen.)
Anyway, long way of saying that I wonder if the mechanism is the same in all cases. It sounds like ANY mechanism will work in a conducting box!