One of the chapters of the book-in-progress talks about neutrino detection, drawing heavily on a forthcoming book I was sent for blurb/review purposes (about which more later). One of the little quirks of the book is that the author regularly referred to physicists trying to “trap” neutrinos. It took me a while to realize that he just meant “detect”– coming from the AMO community, I naturally assume that “trap” means “localize to a small-ish region of space for a long-ish period of time.” That is, after all, what I spent my Ph.D. work doing– trapping cold atoms.
SteelyKid had a rough morning today, so I’m not quite in the right frame of mind for editing this chapter (which is what I really ought to be doing), but I started to make the effort. And immediately got distracted thinking of the “trap” issue. In particular, I made a mention in the text of the several hundred relic neutrinos from the Big Bang believed to be in every cubic centimeter of the universe, phrased in a way that made it sound like they were just sitting there. Which got me wondering what it would take to get neutrinos just sitting still in some region of space.
Of course, a real answer to this question would require me to know a whole bunch of stuff about neutrino physics that I don’t actually know. So in the spirit of students the world over confronted with an exam question they don’t know how to answer, I decided to change the question to something I do know how to attack, namely an estimate of the size of the “trap” you would need to have a neutrino sitting more or less still.
This still seems like an impossible problem, but the key word there is “estimate.” And as long as you don’t want a hard number, I can draw on one of the famous equations that give this blog its name, the Heisenberg Uncertainty Principle:
$latex \Delta x \Delta p \geq \frac{\hbar}{2} $
This says that the product of the uncertainty in the momentum of a particle and the uncertainty in its position must be greater than or equal to a non-zero constant. Thus, it’s impossible to know both of those to arbitrary precision.
The main importance of this is as a concept, rather than something to calculate with, but there is one sort of calculation it’s frequently used for, which is to estimate the properties of a confined particle. If you know that some particle is confined to a region of width $latex \Delta x $, then you know that there must be some uncertainty in its momentum as well. That means you’ll never be sure of finding a trapped particle just sitting still, but you can put a rough limit on the velocity it will have given a particular trapping region. And from that, you can say what the energy of the lowest trap state ought to be, give or take.
So, if we were to confine a neutrino to some region of space, “trapping” it in the AMO sense of the word, what would the velocity be? Because I’m lazy, we’ll use the classical approximation for momentum as just mass times velocity (which isn’t as bad as it might seem, since the goal is to have slow-moving neutrinos, here), and get
$latex (m \Delta v) \Delta x \geq \frac{\hbar}{2} $
$latex v_{min} \approx \frac{\hbar}{2 m \Delta x} $
So, the approximate speed of a trapped neutrino decreases with increasing mass and decreases as you increase the size of the trapping region. Of course, getting an actual number requires a value for the neutrino mass, which we don’t know in an absolute sense. But this is a ballpark kind of calculation, anyway, so we can just pick a value. If we say that our trapped neutrino has a mass of 1 eV/c2 in the units that particle physicists use (a value that’s probably way too big, but convenient), the various constants end up giving you a relationship between approximate velocity in m/s and the “trap” size in meters that’s really simple:
$latex v_{min} \approx 30/\Delta x $
So, a 1eV/c2 neutrino trapped in a 1m box would be moving at an approximate minimum speed of 30m/s. that’s really fast, actually– an electron trapped in the same size box would have a minimum uncertainty-derived speed of about 60 micrometers per second, half a million times smaller.
(As a sanity check, you can ask what this would predict for something like a BEC of atoms, which would be around 100,000 times heavier than an electron (ballpark), in a trap a micron on a side (ballpark), which gets you a minimum speed of about 0.6 mm/s, which is the right general range.)
So, what would it take to get neutrinos “just sitting there?” Well, it depends on your definition. My original phrasing mentioned a volume of one cubic centimeter. If you took that as the trap volume, your neutrinos would be moving at roughly 3000 m/s. If you want them at speeds comparable to the trapped laser-cooled atoms I’m used to, say 0.1 m/s, you would need a trap 300m on a side.
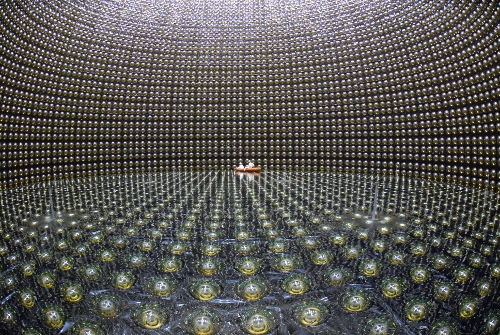
Of course, what you would make the walls of the trap of, in order to confine neutrinos to that volume, I have no idea. Given that you need a 100-m scale tank full of water, like the SuperK detector shown above in the “featured image,” just to have a prayer of detecting a minuscule fraction of the vast number of neutrinos created in the Sun, I don’t think we’ll be actually trapping neutrinos any time soon…
How slow are neutrinos in the wild? My impression is that they are very close to c. Is there any way to get a neutrino to slow down?
If a neutrino has a mass of 1eV/c^2 (which, again, is pretty high), then at 99% of the speed of light, it would have a total energy of around 7eV. The sorts of neutrinos produced in nuclear reactions in the Sun tend to have energies in the keV range, so you’re looking at a lot of nines after the decimal point in the speed as a fraction of the speed of light…
I assume that a neutrino that interacts with a material object could lose energy during the collision, and would thus slow down a bit. Given the tiny mass, the velocity change would be pretty negligible for realistic neutrino energies, but if you could somehow get a single neutrino to scatter many, many (manymanymanymany….) times, you could in principle get it to a very low energy and thus low speed. Of course, I don’t know how you’d manage that, but “in principle” covers all sorts of bizarre crap…
I’m just a simple country molecular biologist, so bear with me. Were there neutrinos produced during the big bang? Are they subject to the same red shift caused by expansion that photons are, and if so, what kind of energies would they have now?
“I’m just a simple country molecular biologist, so bear with me. Were there neutrinos produced during the big bang? Are they subject to the same red shift caused by expansion that photons are, and if so, what kind of energies would they have now?”
EXCELLENT QUESTION!
As it turns out, they were! And they are! And the answer is “not very much at all”.
Cosmic Microwave Background (CMB) photons are microwaves, which peak at about 1mm. So CMB photons all have energy around 1mev, or a milli-electron volt.
The Cosmic Neutrino Background (CNB) was released before the CMB when the universe was in the electro-weak epoch. Once neutrinos decoupled with matter, they were free to propogate just like CMB photons were. So they have even less energy than the CMB.
We have some indirect evidence for the CNB, WMAP claimed they had it to three sigma, but while the CMB is easy to measure due to the high cross section of photons… the CNB can pass through pretty much the entire universe undisturbed.
(This is also why neutrinos are an excellent predictor for supernova. They are released before photons are, so in cataclysmic events, we should see a flux of high energy neutrinos before we see the light)
@Nick: Yes, there were neutrinos produced “during” the Big Bang. More specifically, there would have been neutrinos produced from Z0 decays in the first few microseconds, as well as neutrinos produced in the first hours from neutron decay (800 s half life).
These neutrinos, known as the “cosmological neutrino background” (CNB) are subject to the same redshift as photons.
Because they decouple from matter slightly earlier than photons, they are expected to be _colder_ than the CMB photons, about 1.95 K.
That temperature corresponds to an average energy of 16.8 meV (yes, that’s _milli_ electronvolts). For a 1 eV mass (which is too large, given current limits), the relic neutrinos would have a velocity of 0.09c (p^2 = E^2 – m^2, and v = p/m).
Since the interaction probability (cross section) increases with energy you would have real problems finding walls for your trap at those low energies, indeed. That’s the problem with detecting relic background neutrinos, which we know exist but cannot see 🙂
Why do you assume a box?
Take something the mass of the earth, but not moving, just sitting there really really quietly in intergalactic space. The escape velocity is 11000 metres per second, so Chad’s neutrinos would stick to the planet like glue. The planet could have an essentially unlimited number (limited by some pauli exclusion calculation that I don’t think I want to do) neutrinos hanging around it.
An object like a neutron star near the solar mass limit has about to what it takes to have relic neutrinos trapped around it, out to a 1000 km, depending on that rest mass, but assuming 1 eV mass.
Ha, that’s right! I actually never thought of that. Still, it’s difficult to capture even low energy particles in bound orbits around (or inside) a heavy object, if it doesn’t have repeated interactions. (Compare capture of dark matter particles.) But I’m sure someone has thought of this.
If I could send a detector with really high velocity through the neutrino cloud around a neutron star I could possibly even detect them. Of course that would be difficult in many other ways …
Yes – you would need these low energy neutrinos to have some kind of energy robbing interaction in the neutron star, so that the orbits are not hyperbolic.
Yeah, I’m not a neutrino physicist, but if they start off far from the massive object then they have a lot of potential energy, so they’ll be able to swing in close, speed up, then escape and slow down (without completely stopping) as they fly away.